Spin Angular Momentum
Spin angular momentum is discussed and compared with orbital angular momentum. We explain how spin can be measured via magnetic fields, and explain the issue of chirality and spin.
Angular Momentum, Redux
Like momentum or energy, angular momentum is a conserved quantity in physical systems. It captures the information on how fast an object is spinning, and how hard it is to get it to spin. For particles like atoms, protons or electrons, this comes in two varieties: orbital and spin.
We typically write the former as $\overrightarrow{L}$ and the latter as $\overrightarrow{S}$. Physically, both contribute to the total rotation experienced by a physical system, and it is customary to refer to their sum as
$$\overrightarrow{J} = \overrightarrow{L} + \overrightarrow{S}.$$
Both kinds of angular momentum are subject to the rules of Quantum Mechanics.
A Brief Review of Orbital Angular Momentum
Electrons buzzing around in atoms can have a bit of orbital angular momentum. We discussed it in detail last time. The main idea was that angular momentum necessarily came in integer units of the quantity $\frac{h}{2\pi}$. The faster a particle was rotating, the more units of $\frac{h}{2\pi}$ it had. The moral of the story is that any kind of rotation whatsoever in the macroscopic world is composed of unimaginably many units proportional to $h$.
We also learned a curious connection between the quantization of angular momentum and information: because we can only measure integral units of angular momentum, this put a strict resolution on the observable angle of the direction of that rotation. The faster a given particle rotates - around the proton, say - the sharper the resolution we can observe it.
This was just another example of how $h$ quantizes information in space and time.
Spin Angular Momentum
For macroscopic objects like baseballs, measuring spin is pretty easy. You can mark a position on that ball with a pen, and film it moving. The mark can be used to count the number of times the ball spins in a given time interval. That, together with the size and weight of the ball can be used to compute its angular momentum. For a ball of mass $m$ and radius $r$, we have approximately
$$|\overrightarrow{S}| = \frac{2}{5}mr^{2}\frac{f}{2\pi},$$
where $f$ counts the number of rotations the ball makes per second. In other words, $f$ is the frequency of the rotation.
Like $\overrightarrow{L}$, the direction of $\overrightarrow{S}$ is aligned with the axis of rotation. To specify which orientation is “positive”, we employ the right hand rule: curl the fingers of your right hand about the axis of rotation, and your thumb will point towards the positive direction.
Of course, you cannot draw a dot on an electron or an atom. Macroscopic items like baseballs have a much larger information carrying capacity. To measure a particle's angular momentum we typically need to find something related to it. Typically, we use their magnetic dipole moment.
Magnetic Dipoles and their Moments
Particles carry more than a mass and an electric charge. Some also carry magnetic fields.
Electric charges generate electric fields just by nature of their existence. When electric charges move they also generate magnetic fields. So you might expect a spinning electron to have a tiny magnetic field as well.
Fortunately for us, it does. The proton does too. Lots of particles have tiny magnetic fields. Even some apparently neutral particles like the neutron.
These magnetic fields are directed, like a magnet$^{1}$ or our Earth, they have a “North” and a “South” pole. It just so happens that a particle's dipole magnetic field $\overrightarrow{B}$ is directly proportional to the spin angular momentum:
\begin{equation}\label{b} \overrightarrow{B} \propto \overrightarrow{S}.\end{equation}
This is vector language for saying the the magnitude of the magnetic field is proportional to the magnitude of the spin, and the direction of that magnetic field - the North and South Poles - line up directly with the axis of rotation of that particle$^{2}$.
The dipole magnetic field of particle can be rather complex to describe. It is usually parameterized by a constant vector $\overrightarrow{\mu}$. The direction of $\overrightarrow{\mu}$ defines the direction of $\overrightarrow{B}$, and the strength of $\overrightarrow{B}$ at a distance $\overrightarrow{r}$ is proportional to the magnitude of $\overrightarrow{\mu}$, so that$^{3}$
$$\overrightarrow{B}(\overrightarrow{r}) \sim \mu \left( \frac{3\widehat{r}(\widehat{r}\cdot \widehat{\mu}) - \widehat{\mu}}{r^{3}}\right).$$
These tiny magnetic fields are typically measured using a large, external magnetic field $\overrightarrow{H}$, which induces a torque on the particle to anti-align their magnetic fields. The torque $\tau$ takes a particular simple form using $\overrightarrow{\mu}$
\begin{equation}\label{torque}\overrightarrow{\tau}\; = \overrightarrow{\mu}\times \overrightarrow{H}.\end{equation}
In other words, to measure the spin of an elementary particle, we can measure its dipole magnetic moment, $\overrightarrow{\mu}$.
Chiral Particles and Spin
The spin angular momentum of a quantum particle is a slight refinement of ordinary angular momentum. Spin angular momentum comes in integral units of $\frac{h}{4\pi}$. That extract factor of $\frac{1}{2}$ is related to a deep and complex connection between physics and mathematics, but has an intuitive explanation.
Since $h$ has units of angular momentum, it perhaps makes sense that the smallest value of orbital angular momentum would be a $h$ distributed over the $2\pi$ radians of a full rotation. But some particles come with a slight complication that needs further explaination, they are chiral.
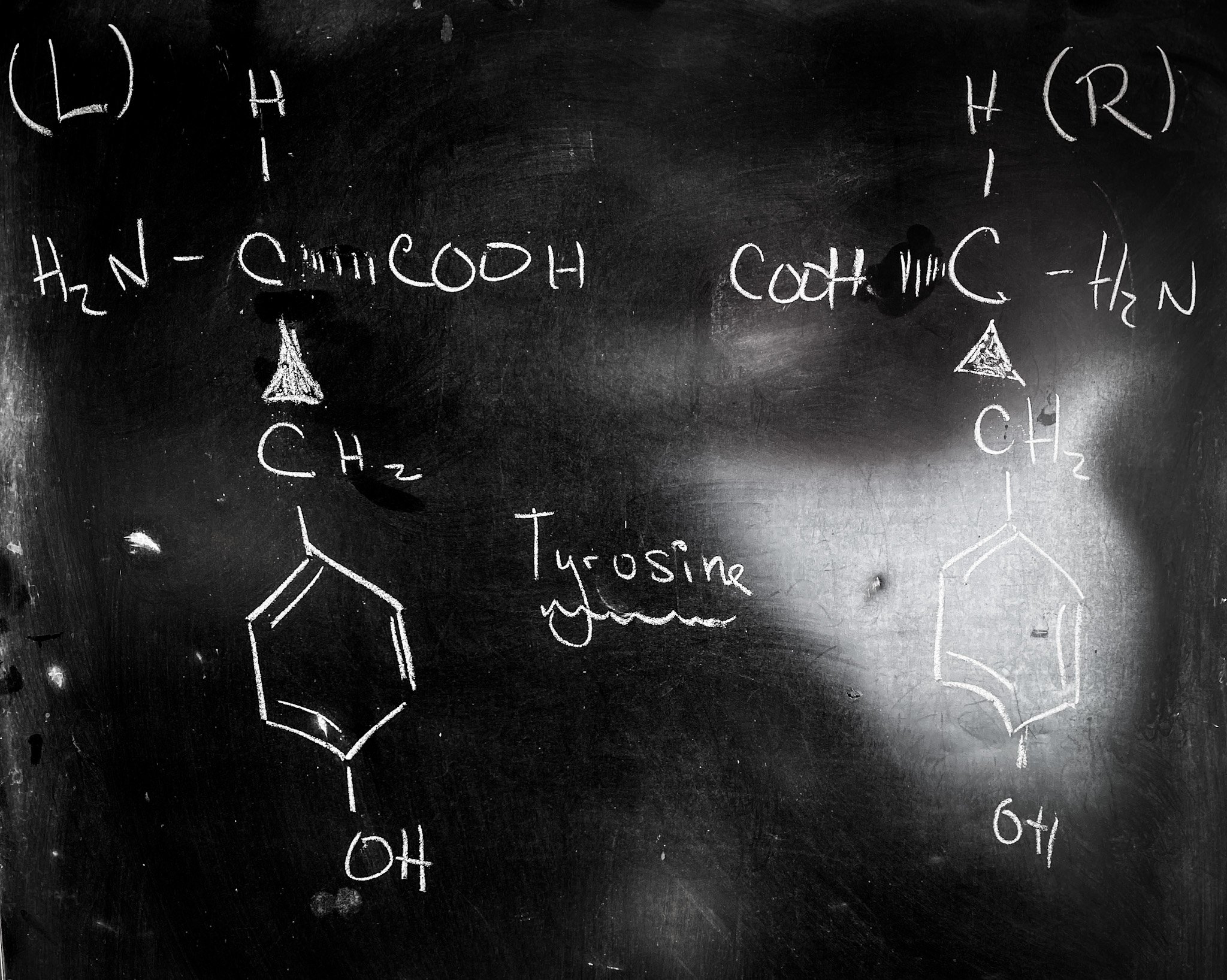
Chiral is just a fancy way to say that something is “handed”. Some molecules are chiral, like amino acids.
Biologically we only used left handed amino acids. Do you know what else is handed? Our hands.
To demonstrate this, place the palm of a hand face up and rotate about a vertical axis normal to its palm. Rotating through $2\pi$ radians, a full revolution, will put that hand and arm in a bit of an awkward spot. If anything this position is uncomfortable, and it certainly isn't back to where it started. To return that hand to its original orientation, you'll need to rotate again by another $2\pi$ radians, for a full, $4\pi$ radian rotation. In other words, chiral or handed things need to rotate through $4\pi$ radians to return to the way they originally where. Thus, when a chiral particle spin about itself - like an electron does - it needs to distribute that $h$ over $4\pi$ radians.
Not all particles are chiral, but many are. The spin of chiral particles comes in units of $\frac{h}{4\pi}$, and the spin of nonchiral particles come in units of $\frac{h}{2\pi}$.
Spin is Fixed
Here are two fun facts about particle spin.
First, the species of particle determines how fast it is spinning. For example, all electrons, all protons and all silver atoms always have a magnitude of spin angular momentum equal to
$$|\overrightarrow{S}_{e}| = \frac{h}{4\pi}.$$
Similarly all pions have no spin angular momentum at all. Photons are not chiral particles, and all photons have a magnitude of spin angular momentum
$$|\overrightarrow{S}_{\gamma}| = \frac{h}{2\pi}.$$
Second, all particles are either chiral or not chiral, and that too depends on their species. In particular, a chiral particle will always be observed to be chiral. What this means is that chirality imposes similar constraints on the angular resolution for measuring the direction of a particle's spin angular momentum. In particular, an electron, whose spin angular momentum is $\frac{h}{4\pi}$ can only ever be measured as
$$ \overrightarrow{S} = \frac{h}{4\pi}\;\mathrm{or}\; -\frac{h}{4\pi}.$$
This corresponds to measuring the direction of $\overrightarrow{S}$ at $0^{\circ}$ or $180^{\circ}$.
Similarly, a deuteron - the nucleus of an $^{2}$H atom - has a spin of $|\overrightarrow{S}| = \frac{h}{2\pi}$, which only ever be measured as
$$ \overrightarrow{S} = \frac{h}{2\pi},\;0,\;-\frac{h}{2\pi}.$$
Like the $p$-orbitals from our discussion of orbital angular momentum, This corresponds to measuring the direction of $\overrightarrow{S}$ at $0^{\circ}$, $90^{\circ}$ or $180^{\circ}$.
Similarly, a $\Delta$-baryon, which has a spin angular momentum of $|\overrightarrow{S}| = \frac{3h}{4\pi}$, can only ever be measured as
$$ \overrightarrow{S} = \frac{3h}{4\pi},\;\frac{h}{4\pi},\;-\frac{h}{4\pi}\; \mathrm{or} -\frac{3h}{4\pi}.$$
Another way to think of chirality is that chiral particles are always observed to be an odd integral unit of $\frac{h}{4\pi}$, and achiral particles are always observed to have even integral units of $\frac{h}{4\pi}$.
Exercises
Exercise 1 : In the presence of a magnetic field $\overrightarrow{B}$, particles with a magnetic moment $\overrightarrow{\mu}$ experience a torque. The magnitude of the torque is proportional to the angle between the particle's magnetic moment and the magnetic field:
$$\tau = \mu B \sin\theta.$$
If $\overrightarrow{B}$ is large enough, this torque causes the particle with magnetic moment $\overrightarrow{\mu}$ to align with $\overrightarrow{B}$.
This can be recast as a potential energy
$$U = -\overrightarrow{\mu}\cdot \overrightarrow{B},$$
which is minimized when the two are aligned.
As with everything in the atomic realm, photons can be absorbed or emitted to change the energy of the particle.
Let $S$ be the magnitude of that particle's spin angular momentum. What wavelengths of light can be observed if we subject a collection of such particles to a strong magnetic field $\overrightarrow{B}$?
Exercise 2 : We've studied the electron orbiting the hydrogen atom intensely. We now know that it can have angular momentum of both the spin and orbital variety.
Let
$$\overrightarrow{J} = \overrightarrow{L} + \overrightarrow{S},$$
be the sum of the two. For an electron excited into the $p$-orbital with one unit of angular momentum, what are the total observable values of $|\overrightarrow{J}|$?
$^{1}$ : In a very real sense, these tiny, atomic magnetic fields in metals like iron all line up to form the magnets we use in daily life. More on this curious fact in a future episode.
$^{2}$ : A practicing physicist would probably take umbrage with that framing. Just like we can't draw a point on an electron, we can't really define the shape or size of it either. So it doesn't really make sense to define an axis of rotation. In practice, physicists simply treat spin as an inherent property of a particle.
$^{3}$ : Here vectors with a hat, like $\widehat{\mu}$ are unit vectors. They have magnitude 1, and so only really represent a direction. We can write, $\overrightarrow{r} =|\overrightarrow{r}|\widehat{r} = r\widehat{r}$, for instance.
