The Stern-Gerlach Apparatus
Quantum Mechanics can be distilled into two major themes.
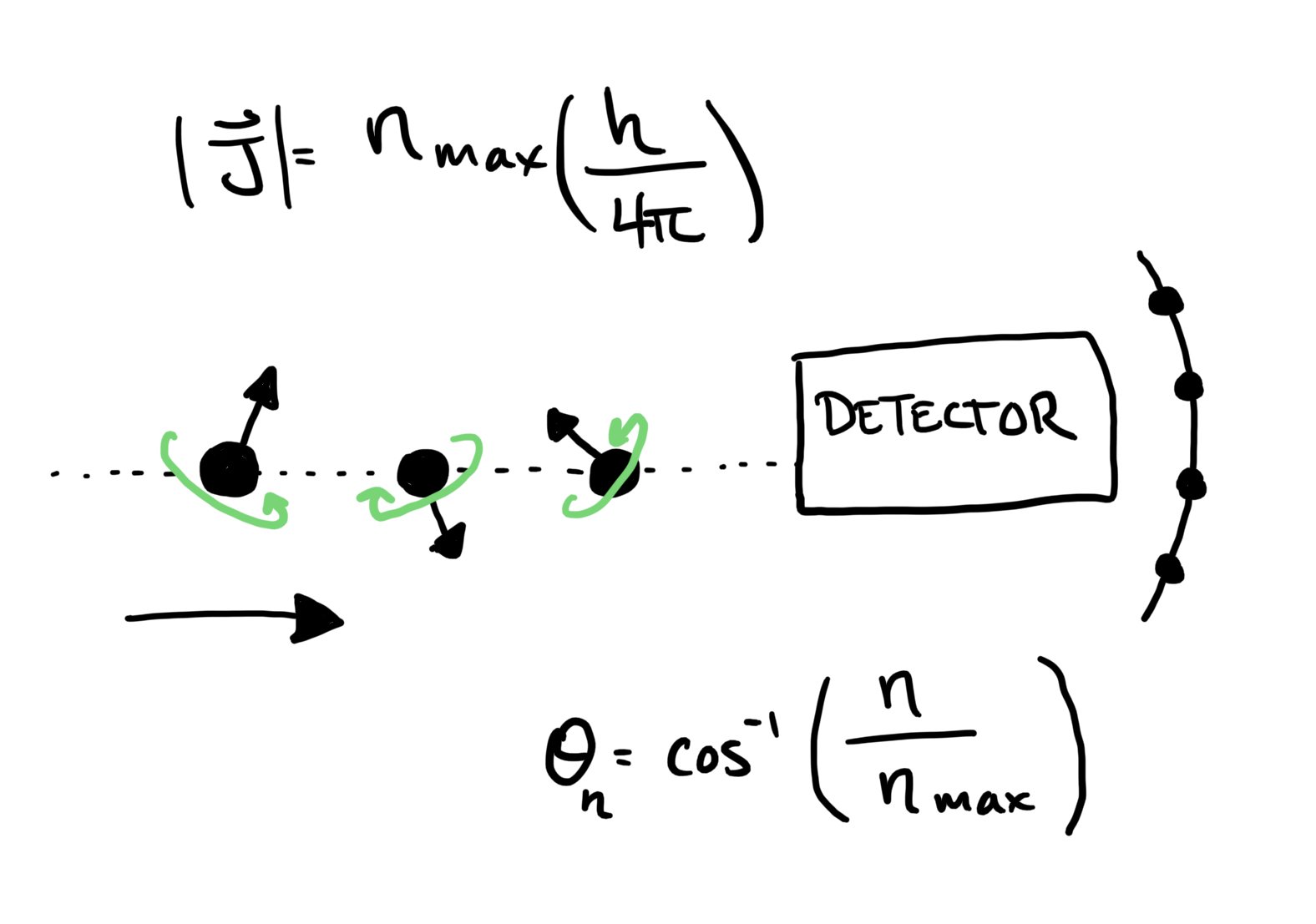
The first is Planck’s constant, $h$. It represents a fundamental resolution for the information carrying capacity of space and time. We’ve studied at length how, for example, $h$ restricts the number of angles we can observe in studying an atom or molecule’s angular momentum.
For the simplest case of say, the spin angular momentum of silver atoms, electrons or protons, there are only two angles that can be observed: 0 and 180 degrees. These corresponding to values of that angular momentum of $h/4\pi$ or $-h/4\pi$. Some folks call these spin up and spin down, respectively$^{1}$.
The second major theme is probability. Just because you observe a silver atom with a spin aligned or anti aligned with a device, doesn’t mean that is what it’s spin angular momentum actually was. It could have been spinning in any direction, at any angle with respect to the device. Morally, the likelihood of observing the spin in the up or down orientation is set up so that the average value$^{2}$ is given by the cosine of the angle between them:
\begin{equation}\label{counts}\frac{N_{\sf up} - N_{\sf down}}{N_{\sf up} + N_{\sf down}} \rightarrow \cos\theta.\end{equation}
But the fact is, any time you ask nature the question, you’ll only get the discrete answer.
A clarifying complaint
Naïvely, these two points seem to contradict each other. You might complain:
Doesn’t this probabilistic feature of quantum mechanics mean that the universe can and does carry information, it’s just that we cannot access it? I mean, if the actual angle influences the probability distribution, doesn’t the universe know what the correct angle is?
Excellent question! This question is at the heart of understanding how Quantum Mechanics works. Suffice it to say, the answer always comes from experiment.
This is what Science is.
Information is literally made by experimental observation. And experiment says that you cannot predict what is going to happen - beyond specifying the relative probabilities. Hence. Nature cannot communicate that information with perfect fidelity. But it can with some fuzziness. That’s what we mean by a finite resolution of information.
We will come back to this conflict of ideas in due course. But for now, let’s look at the first experiment that verified this phenomenon in spin angular momentum: the Stern-Gerlach Experiment.
The Stern-Gerlach Apparatus
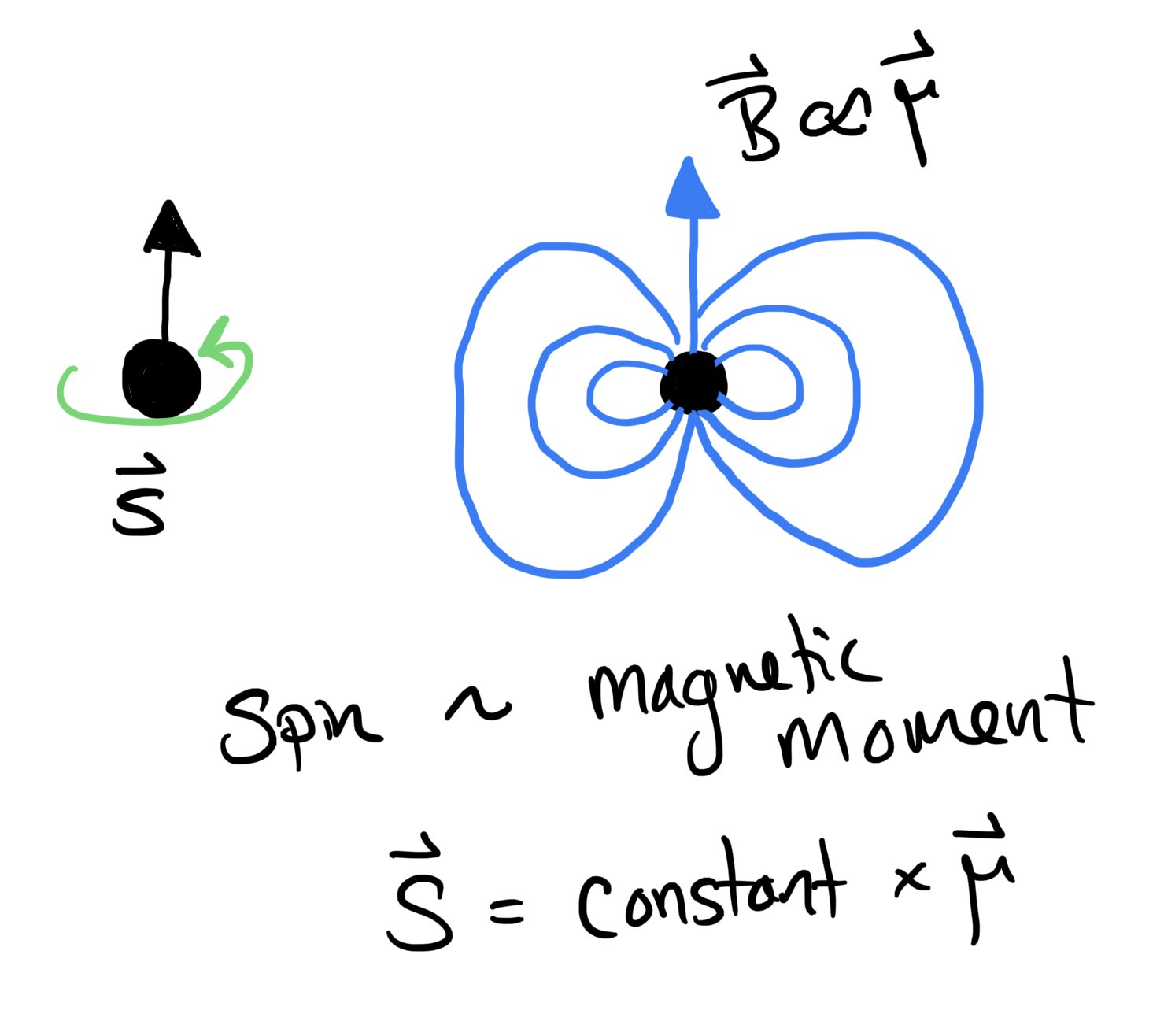
Last time we learned that spin angular momentum sometimes bestows a tiny magnetic field to a given particle. Sometimes there is a clear reason: if the particle is electrically charged, motion will certainly generate a magnetic field. Sometimes it is less so: the electrically neutral neutron has a tiny magnetic field. In this latter case what’s actually happening is that the neutron itself is comprised of other particles, which themselves have an electric charge. The neutron’s spin is an amalgam of theirs, and so it gets its own magnetic field. This is handy because it’s the one way we can interact with neutrons. We can use magnetic fields!
Atoms too can have tiny magnetic fields associated to their spin. They are bound states of positively charged nuclei and negatively charged electrons. The silver atom is a particularly nice atom to consider because its net spin angular momentum is precisely the same as a proton or a neutron: $h/4\pi$. Therefore, it has precisely two angles that we measure it at, corresponding to the spin up or spin down values of plus or minus $h/4\pi$, respectively. It’s also electrically neutral, so we don’t have worry about those kinds of interactions. The Stern-Gerlach experiment involved shooting a beam of silver atoms, through a magnetic field, and into a detector.
Let’s sketch how it worked.
The Stern-Gerlach Experiment
There are three parts:
The Beam
The magnetic field which separates the beam based on its magnetic field.
The detector screen.
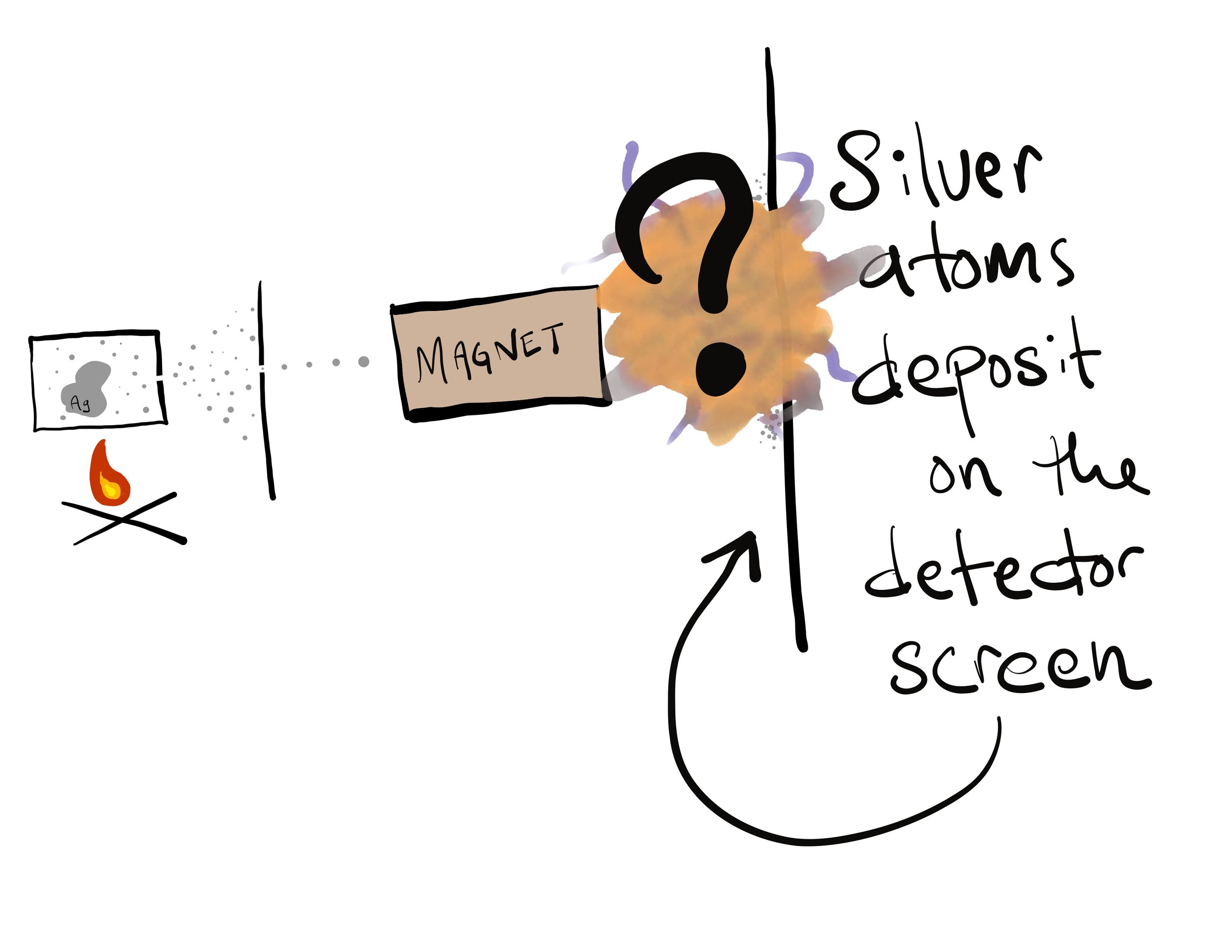
If what Quantum Mechanics tells us is correct: that we can only measure two angles of a silver atom’s spin angular momentum, the magnetic field which separates the beam will split it in two.
Upwards for spin up. Downwards for spin down. If Quantum Mechanics isn’t real, then the beam will be smeared instead, depending upon the angle between the spin of the silver atom and the magnetic field of the device. We’ll be able to tell what happens by looking at how the silver atoms despite on the detector screen at the far end of the experiment.
Let’s now visit each of these components separately.
The Beam
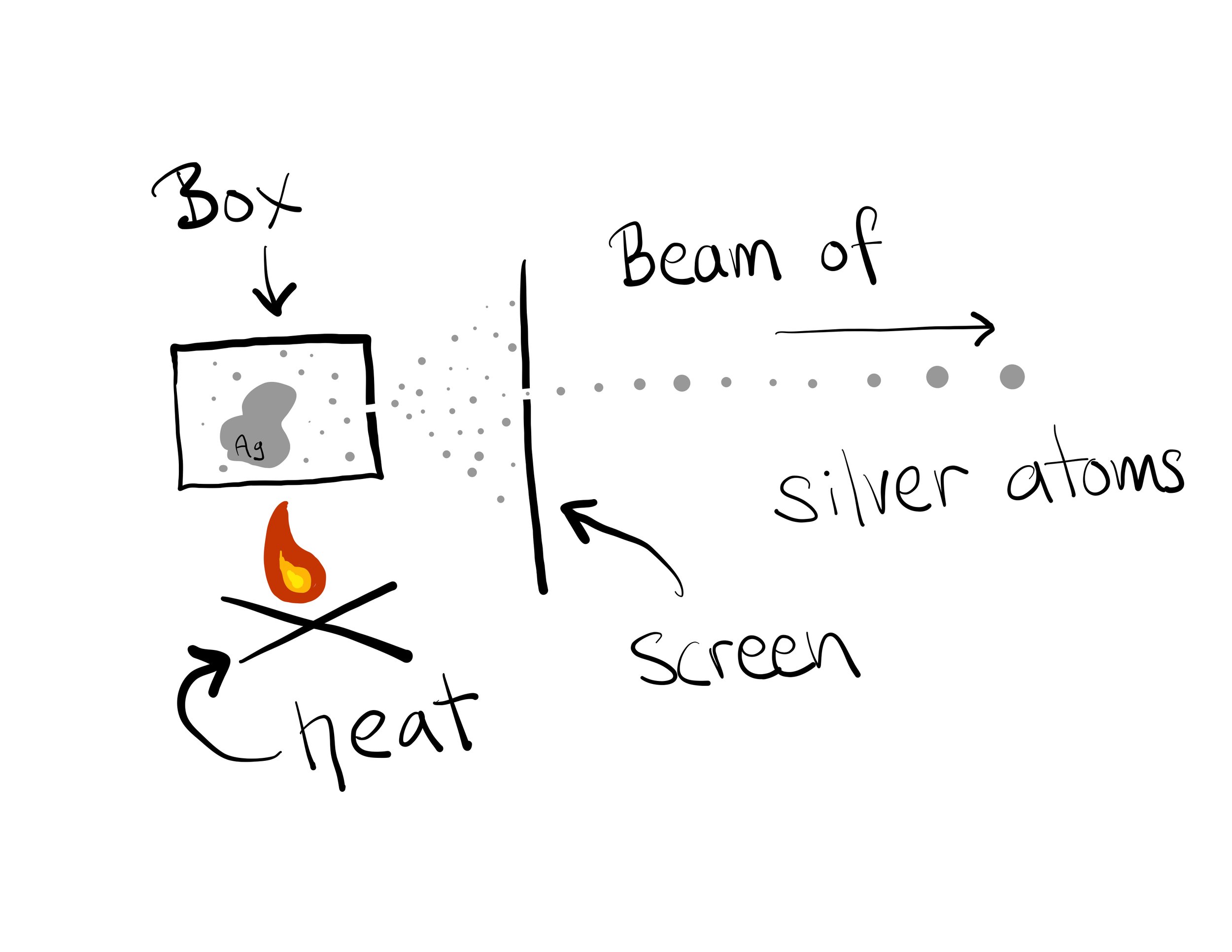
Take a lump of silver and place it in a box. Heat the box so that some of the silver atoms sublimate off from the lump. Next poke a tiny hole in the box. This will cause a little spray of silver atoms to come out at random, whenever they happens to pass through the hole.
To create a beam out of this spray, you can put another screen further out, and poke a hole through it. That way, only the atoms that came through the hole in precisely the right way continue pass the screen. It’s not very efficient, obviously, but there are a lot of silver atoms in a lump of silver. This is a quick and easy way to create a beam of silver atoms, with technology that was certainly available in the 1920’s.
Now we aim that beam at a magnetic field.
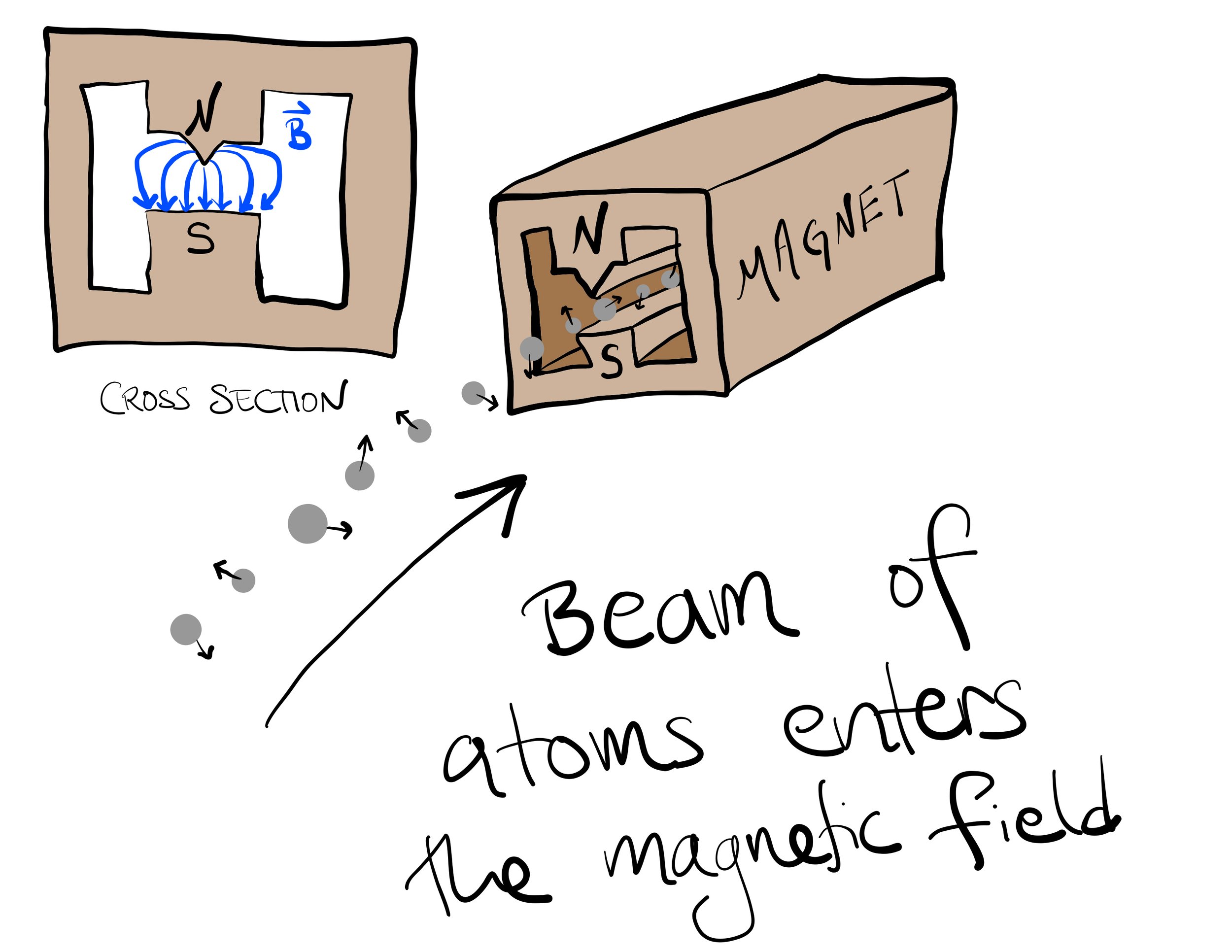
The Magnet
Last time we learned that the magnetic moment $\overrightarrow{\mu}$ of a spinning particle is directly proportional to its spin angular momentum.That particle then experiences a torque
$$\overrightarrow{\tau} = \overrightarrow{\mu}\times \overrightarrow{B}.$$
in the presence of a constant magnetic field $\overrightarrow{B}$.
That torque is associated to a potential energy $U$:
\begin{equation}\label{U}U = - \overrightarrow{\mu} \cdot \overrightarrow{B} = -\mu B \cos\theta.\end{equation}
The potential energy is minimized - that is, its lowest value occurs - when $\theta = 0$, that is, $\overrightarrow{\mu}$ and $\overrightarrow{B}$ are precisely aligned. Otherwise, there a torque$^{3}$.
The above formula for $\tau$ is actually specific to a constant magnetic field, but the formula for the potential energy $\eqref{U}$ is exact. In particular, \eqref{U} holds even when $\overrightarrow{B}$ has a crazy complicated shape.
You might recall from elementary physics that a force $F$ is related to a potential energy as
\begin{equation}\label{f}\overrightarrow{F} = -\overrightarrow{\nabla}U.\end{equation}
That is, a conservative force is associated to the gradient$^{4}$ of a potential energy. If the magnetic field varies in space, the little silver atom will actually experience a force when passing near that changing magnetic field.
In short, Stern and Gerlach took a big magnet with a sharply pointed end. So that it had a large derivative in the X direction. Plugging \eqref{U} into \eqref{f}, we find that the silver atoms will experience a force
$$\overrightarrow{F} = \mu \frac{dB}{dx} \cos\theta.$$
A spatially varying magnetic field can kick a particle with a magnetic moment. Remember that the kick depends on the direction in which the magnetic field changes, not necessarily the magnetic field itself!
For our purposes today, it doesn’t matter what $dB/dx$ is. What matters is that the force is proportional to it, and that it’s big enough to give those atoms a kick. A sharply pointed end will do just that! As you can see, it also depends on the angle between the magnetic moment - that is, the angle between the detector and the spin.
In other words, the silver atoms will float along in their beam until they approach the magnet. Then they’ll experience a force promotional to the cosine of the angle at which they’re rotating with respect to the detector. The magnet will smear out the beam.
The Detector
The silver atoms are coming out of the oven at random times, spinning in a random directions. If Quantum Mechanics is right, there are only two observable angles, $\theta = 0^{\circ}$ and $180^{\circ}$. This means that there are only two values of the force from \eqref{f}:
$$F = \pm \mu \frac{dB}{dx}.$$
Because it restricts the angular resolution of the spinning particles, Quantum Mechanics suggests that the magnet will split the beam in two. If Quantum Mechanics is wrong, all angles could be present, and the beam will smear, per $\eqref{f}$.
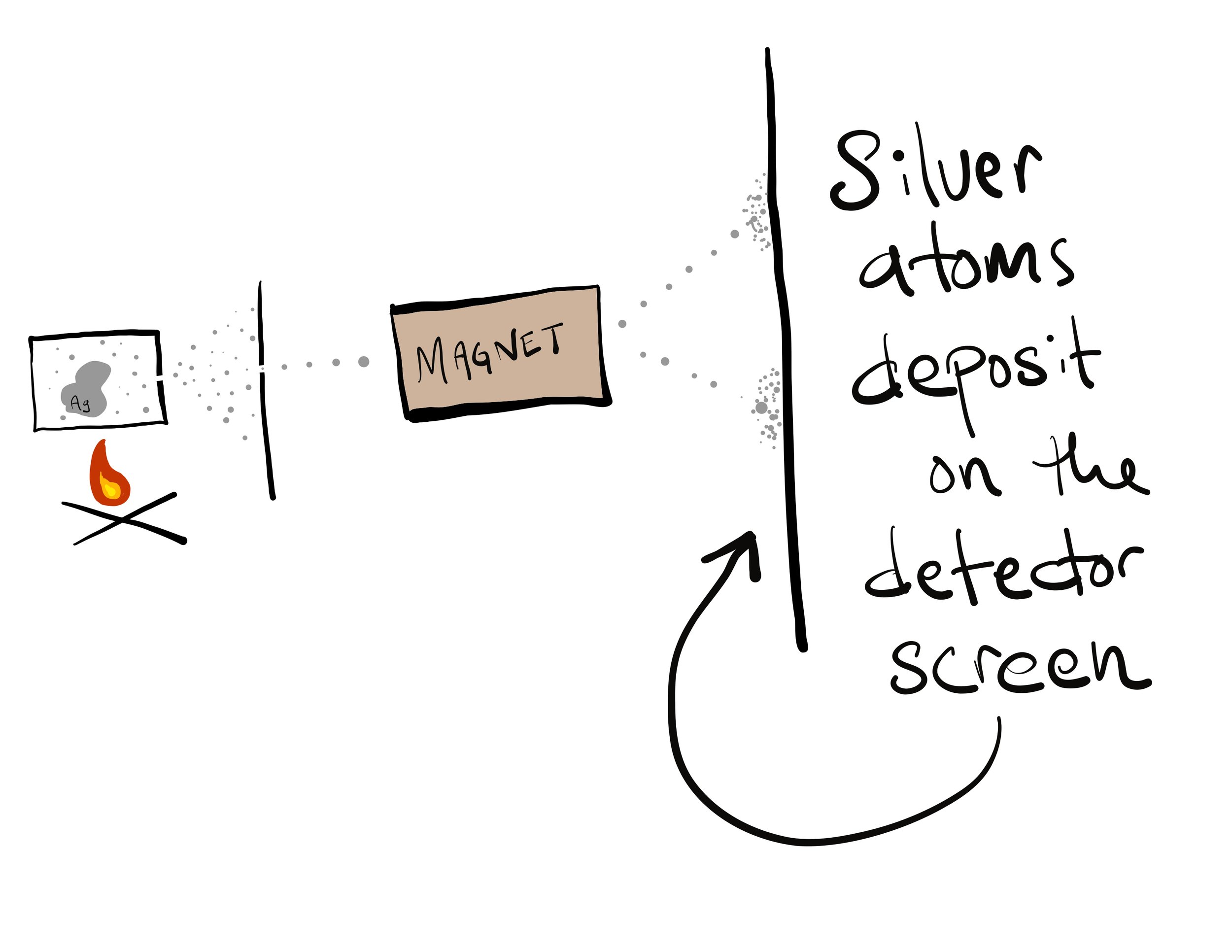
Once past the magnetic field, the silver atoms will then deposit themselves on the screen. Looking for the silver atoms on the other end of the devicve, we can see how they moved past the magnet. Did the beam split in two? Or did the beam smear out? Or did something else happen?
The Experimental Result
The beam split in two, of course. If it hadn’t we wouldn’t be here talking about Quantum Mechanics.
If instead Stern and Gerlach had used an electrically neutral particle with spin angular momentum $h/2\pi$, we’d see the beam split in three. If it was $3h/4\pi$ we’d see it split in four.
Classical objects like balls have a very high angular resolution because they are made up of a ton of tiny little particles. That’s the difference between the quantum description of atoms and the classical description of our usual reality.
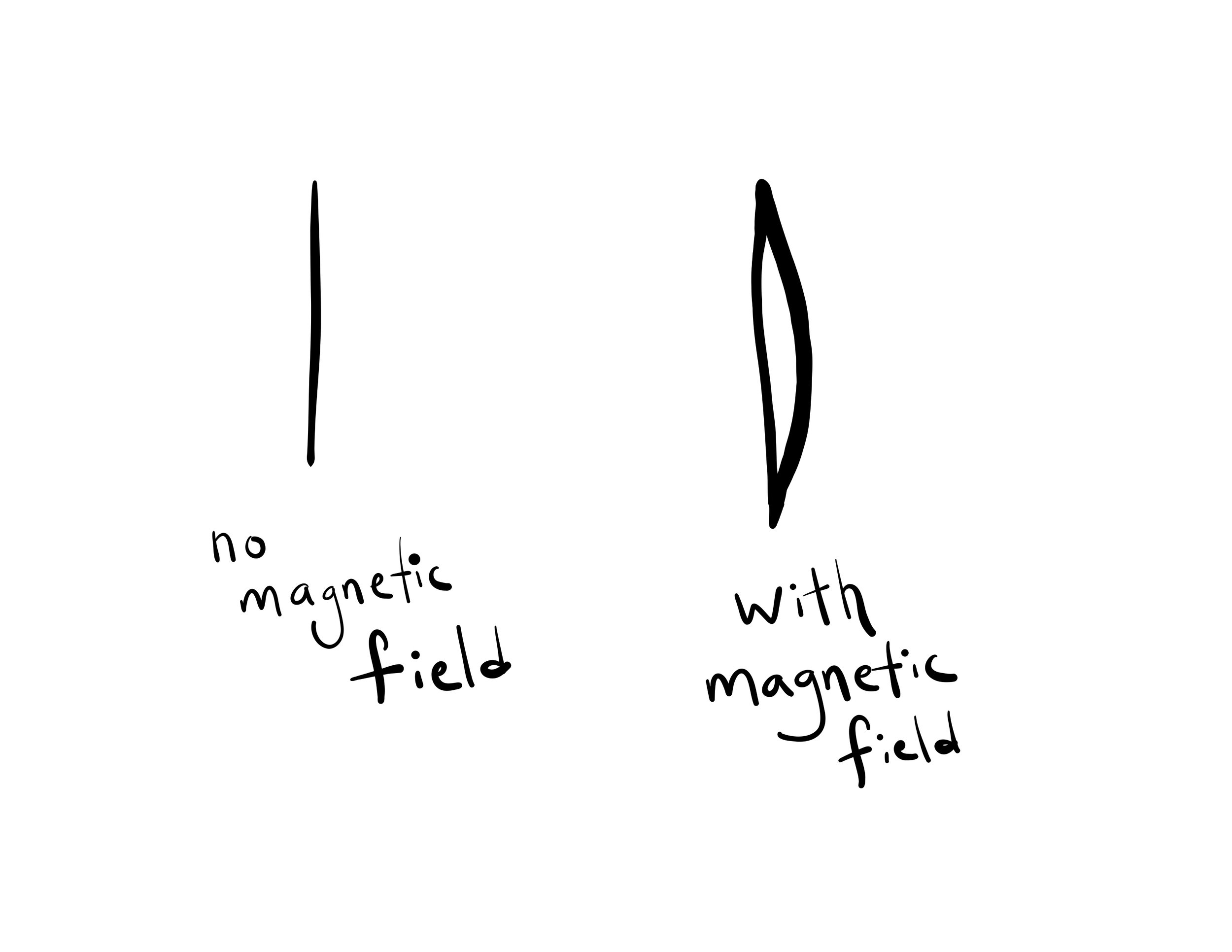
It’s worth showing you exactly what they did see, which happened to be replicated on a postcard. It’s helpful because its the actual evidence and it demonstrates how to interpret experimental results in terms of the nice, clean models we’ve been discussing.
A replica sketch of the silver deposits placed on the detector screen in the original Stern-Gerlach experiment. These kinds of images were sent, for example, on a postcard to Niels Bohr shortly after the experiment concluded. Copies of it abound, for example, see this paper, or consult the Niels Bohr archive.
The observational signature - what they actually saw on the detector screen - looked more like an oval than a pair of dots. What accounts for this?
Well the thickness of the lines is clearly related some of the uncertainty involved with where the silver atoms landed. It’s related to the thickness of the beam itself. Some of this could be having to smash through air molecules. Some of it could be related to size of the holes. Often we call this kind of thing experimental noise. Atoms in a beam with a little thickness to it will recieve differential kicks from the magnet$^{5}$, exacerbating that noise as the beam split.
Atoms in the beam that didn’t get close enough to the magnetic field gradient didn’t get kicked as hard. Those that lined right up on it got kicked - and therefore separated - the most. Eventually that kick became so modest that it couldn’t be distinguished from the noise associated to the atoms moving down the beam line, which explains why it pinches off near the end.
What’s important is that at the center - where the impact of the magnetic field is the strongest - you can clearly see the beam split in two. There’s nothing in the middle. If quantum mechanics hadn’t work out the way it did, instead of oval like shape outline, you’d have a filled in blob.
Exercises
Exercise 1 : Derive the result \eqref{counts}. You may wish to refer to the past two lectures. Explain when it is valid.
Exercise 2 : Argue why the silver atoms are coming out of the oven at random times, spinning in a random direction.
Exercise 3 : If all angles could be observed, what would the distribution of silver atoms look like on the detector screen? In particular, if we had an atom with a spin angular momentum of $ 15 h/4\pi$, what, precisely, would you expect to see?
$^{1}$ : Up and down with respect to what? With respect to the axis of the detector.
$^{2}$ : More precisely, we say that the expectation value of the underlying probability distribution is given by $\cos\theta$.
$^{3}$ : Note that $\cos\theta$ also vanishes when $\theta = 180^{\circ}$. That is, when the two are antialigned, and the potential energy is maximized. This is a bit like a ball resting precariously at the top of a hill. It’s an unstable solution. Even a little deviation from $180^{\circ}$ will induce a torque. Ironically, $0^{\circ}$ and $180^{\circ}$ are the only two angles that are observable for a silver atom. While we won’t consider the case of constant $\overrightarrow{B}$ much today, we’ll come back to this observation later in our study of the Ising Model.
$^{4}$ : The gradient measures how quickly a function is changing. More precisely, it is the directional derivative of a function. For $U$, that is
$$\overrightarrow{\nabla}U(x,y,z) = \frac{\partial U}{\partial x}\widehat{x} + \frac{\partial U}{\partial y}\widehat{y} + \frac{\partial U}{\partial z}\widehat{z}.$$
As this is not a math course, we won’t worry too much about the notational distinction between partial and regular derivatives unless we need to.
$^{5}$ : A sharply pointed magnet will have large $dB/dx$, and will also likely have a nonzero $d^{B}/dx^{2}$, suggesting that atoms approaching the magnet from slightly different distances, as in a thickened beam, will receive slightly different kicks. Of course, good experimental practice would be to try to keep $B$ as linear in $x$ as possible. But the sharp point cannot extend forever. The finite size of the magnet will eventually have some effect on the beam.
