The Scales of Quantum Phenomena
Quantum Mechanics modifies laws of Physics at microscopic scales. We in- terrogate the meaning of “microscopic” in the context of Coulomb’s Law for the Electric force.
The Scale of Physical Models
Quantum Mechanics describes physics on microscopic scales. Its effects deviate from the physical models we normally use to describe our everyday experience. There are some striking differences, and many subtle points. Before we get into these technical discussions, we should probably ask:
What exactly do we mean by “microscopic”?
Physical models are often constrained by parameters. Let’s consider an old, familiar example: Gravitation.
The motion of the planets in our Solar System can be modeled by Newton’s universal gravitation force. This force depends the masses of the objects involved and, importantly, the universal gravitational constant, $G$:
\begin{equation}\label{gravity}\overrightarrow{F} = -G\frac{Mm}{r^{2}}\widehat{r}.\end{equation}
This weakness is manifest in the value of G:
$$G = 6.67430(15) \times 10^{-11} \; \mathrm{J}\cdot \mathrm{m} \cdot \mathrm{kg}^{-2}.$$
Gravity is a very weak force, so even enormous objects like planets and moons orbit each other at distances measured in the hundreds of thousands to hundreds of millions of miles.
Electromagnetism, by contrast, is a strong force. Coulomb’s Law has an analogous model for the force:
\begin{equation}\label{em}\overrightarrow{F} = -\frac{1}{4\pi\epsilon_{0}}\frac{e^{2}}{r^{2}}\widehat{r}.\end{equation}
The analogous force parameter certainly appears larger:
$$\frac{1}{4\pi\epsilon_{0}} = 8.98755179 \times 10^{9}\; \mathrm{J}\cdot \mathrm{m} \cdot \mathrm{C}^{-2},$$
although these two aren’t a priori comparable as they have different units. Gravity acts on masses, the electric force acts on charges. To realistically compare them, we need to consider things that have both a mass and a charge.
The Force that Binds Atoms
Atoms are comprised of protons and electrons. Both particles have an electric charge and a mass. For the scale of a simple hydrogen atom: one proton with one electron, we can compare the strength of gravity to that of electricity.
Protons have a mass of around $1.67 \times 10^{-27}$ kg and a positive electric charge of about $1.60 \times 10^{-19}$ C. Electrons have a much smaller mass, around $9.10\times 10^{-31}$ kg, but an equal yet opposite electric charge. Therefore both particles are subject to both gravity \eqref{gravity} and Coulomb's Law \eqref{em}:
$$\overrightarrow{F} = \overrightarrow{F}_{G} + \overrightarrow{F}_{E}.$$
Plugging their masses and charges into \eqref{gravity} and \eqref{em}, we find that
\begin{equation}\label{compared}|\overrightarrow{F}_{G}| \approx -\frac{1.01 \times 10^{-72}}{r^{2}} \; \mathrm{N},\quad |\overrightarrow{F}_{E}|\approx -\frac{2.30 \times 10^{-28} }{r^{2}} \; \mathrm{N}.\end{equation}
In short, the total force$^{1}$ is almost completely dominated by Coulomb's Law.
Looking at the differences in \eqref{compared}, it becomes easy to understand why gravity binds objects with orbital lengths well into the hundreds of thousands of miles. Whereas, the size of a typical atom is measured in Ångströms: [$10^{-10}$ m].
Ångströms are the scale where we’ll begin explore Quantum Mechanics, but it's worth noting that quantum effects can occur on any length scale.
Planck’s Constant and the Scale of Quantum Mechanics
We can learn about the scale quantum theory by investigating its fundamental parameter known as Planck's constant, $h$.
The rôle of h is set a fundamental resolution for information density in space and time.
On average, the corrections to classical physics from quantum theory can be expressed in terms of powers of $h$. Taking $h\rightarrow 0$ means that we assume that every point in spacetime has an infinite capacity to store information. This limit - at least, when it is well-defined - takes us back to the classical realm familiar from elementary Physics.
Sometimes you cannot consistently take the limit h → 0. Lasers are a great example. Clearly, we will be very interested in these exceptional cases.
By itself, the exact value of $h$ isn't terribly illuminating.
$$h = 6.62607015 \times 10^{-34}\, \mathrm{J}\cdot\mathrm{s}. $$
To build a better intuition for $h$ in the context of Chemistry, it helps to incorporate another fundamental constant of nature.
The speed of light, $c$, sets a universal limit on the rate at which that information can travel.
It's just shy of $3\times10^{8}$ meters per second,
$$c = 299,792,458 \,\mathrm{m}/\mathrm{s}.$$
$c$ has units of velocity, befitting the flow of information through space. Note also that because $c$ is used to define what a meter is, so this definition of $c$ is exact. But it’s still wonky, and difficult to appreciate alone. It’s big, sure, but so what?
A Useful Combination
Multiplied together, the combined value $hc$ is often more useful in practice. It has a value$^{2}$ of
\begin{equation}\label{hc1} hc \approx 1240\, \mathrm{eV} \cdot \mathrm{nm}.\end{equation}
Because h sets the scale for quantum phenomena, this combination will be the “ruler” or “scale” at which we can judge whether quantum effects are important or not.
In this form, $hc$ associates a “quantum” or “microscopic” length scale to any given energy - or vice versa. Let $E$ be some known energy - perhaps the energy a proton in an electric field. Then to any such $E$ we can associate a length $\ell_{E}$ by simply taking a ratio:
$$\ell_{E} = \frac{hc}{E}.$$
As we shall see, physics at any length smaller than $\ell_{E}$ will be impacted by corrections from Quantum Mechanics.
Alternatively, given a characteristic length scale $\ell$ - like the size of some given atom - we can associated a ``microscopic'' energy $E_{\ell}$ by a similar ratio:
$$E_{\ell} = \frac{hc}{\ell}.$$
Similarly, the laws of physics operating at an energy lower than this will be impacted by Quantum Mechanics.
If $xE \gg$ 1240 eV$\cdot$nm, then no. We probably don’t have to worry about Quantum Mechanics. But if $xE \sim$ 1240 eV nm. We probably should look more carefully.
Atoms, for example
The units involved with $hc$ in \eqref{hc1} suggests that Quantum Mechanics becomes important to consider at the nanometer scale. An important example of physics on the scale of nanometers is light itself!
The colors of visible light span a range from about 400 nm (violet) to 700 nm (red). The energy scale related by $hc$ to these wavelengths of light are just a few eV.
In practice, this is just shy of the energy required to rip an electron from an atom - or even to break most molecules apart. $hc$ tells us that quantum effects associated with visible light should have something to do atomic physics.
As we shall explore in the coming lessons, there is a deep relationship between visible light and the dynamics of electrons inside the atom.
Atomic nuclei, for another example
The value of $hc$ given in \eqref{hc1} can be expressed in different units,
$$ hc \approx 1240 \;\mathrm{MeV}\cdot\mathrm{fm},$$
Here the fermi or femtometer [fm] is given by $10^{-15}$ meters. A typical atomic nucleus is about 1 fm across.
As above, the average binding energy between atoms in molecules is around 1 to 10 eV. This sets the scale for molecular or electronic physics. By comparison, the average binding energy between protons and neutrons in the nucleus is between 1 to 10 MeV.
This corresponds to the femtometers scale, which we have observed to be the scale of nuclear physics.
In short, the phenomenology of quantum effects associated to the nuclear interaction essentially mirror those of the electronic interactions at this much, smaller scale.
The Hydrogen Atom
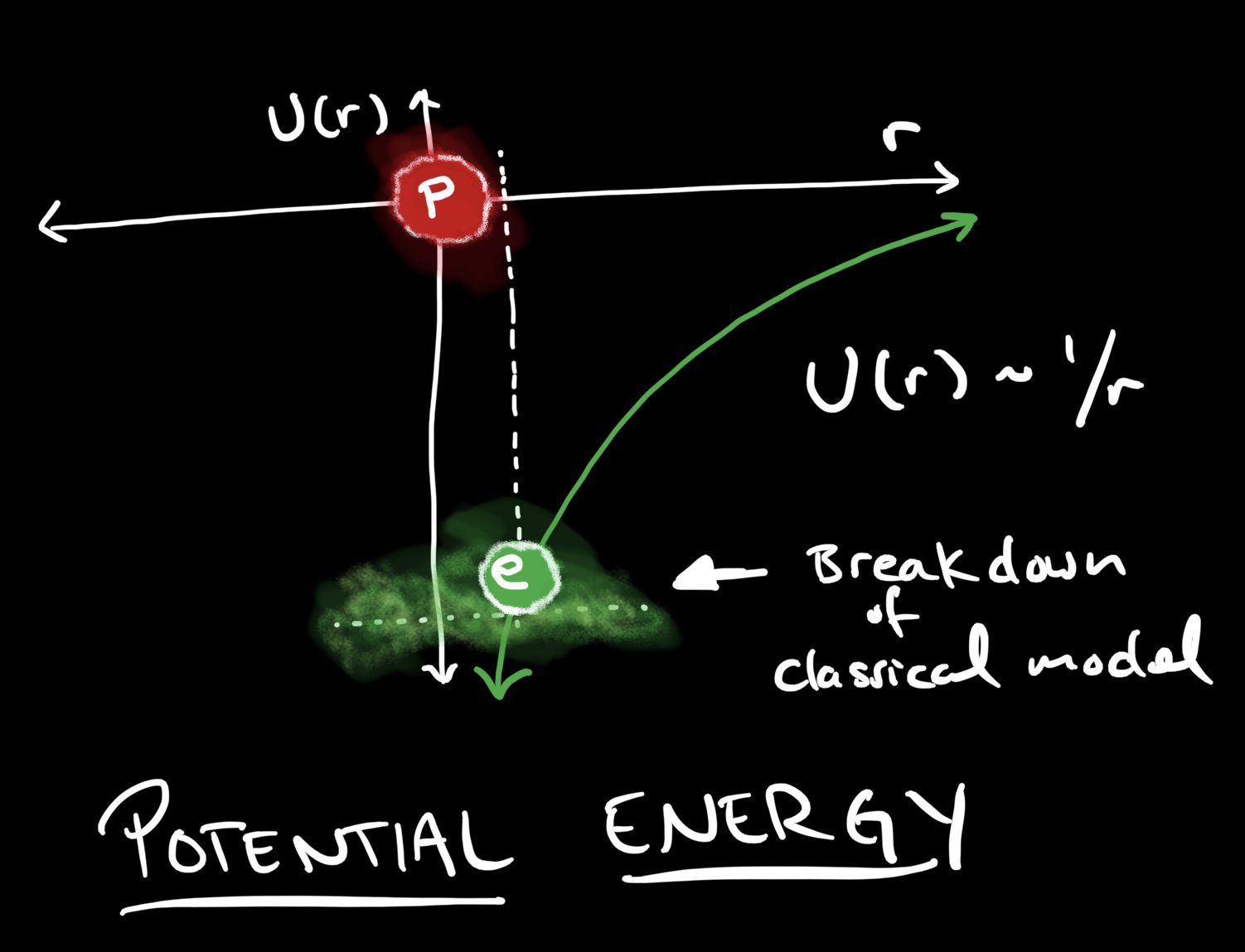
To think about the scale of quantum mechanics in electromagnetism, it helps to think of the electric force \eqref{em} as a potential energy$^{3}$:
\begin{equation}\label{u}U(r) = -\frac{e^{2}}{4\pi\epsilon_{0}r}.\end{equation}
The proton is heavy and sources an electric field. The electron is light and, having the opposite charge, is attracted to the proton. The mathematics involve is just a simple integral with some careful choice of boundary conditions$^{4}$, but essentially the electron experiences the electric force as a potential energy “well” or “sink” which it can fall into.
By itself, this suggests that the electron should fall all the way down the well, and slam into the proton. But we know atoms are stable. At some value of r, our model for the electric force between the proton and the electron breaks down.
How it breaks down is a question for Quantum Mechanics, and understanding the “how” is our goal for this course. But first, let’s ask an easier question: Where does it break down? At what value of r?
To get a sense, let’s evaluate \eqref{u} with what we know: that the electron orbits at around 1Å:
$$U(1Å) \approx 2.3 \times 10^{-18} \;\mathrm{J} \approx 14 \;\mathrm{eV}.$$
This is astonishingly close to the actual value of 13.6 eV! Given this value of Energy, let’s take a look at what length scale corresponds to our threshold for Quantum Mechanics \eqref{hc1}:
$$\ell_{H} = \frac{1240\;\mathrm{eV}\cdot\mathrm{nm}}{14\;\mathrm{eV}}\approx 88.5 \;\mathrm{nm}.$$
This is much larger$^{5}$ than 1Å!
So, from the perspective of potential energy - derived from Coulomb’s Law - Quantum Mechanics becomes important to describe the physics of the electron at much greater distances than the observed atomic radius.
Indeed, the atom does not collapse because of quantum effects. The electron does not fall down the well in \eqref{u}. The breakdown of our models - our use of Newtonian physics with the conservative potential energy \eqref{u} occurs well before the electron reaches its final resting radius near 1Å.
Exercises
Exercise 1 (Calculus Based) : Derive the Coulomb potential (5) from the Coulomb force (2) using the formula:
$$ U(r) = -\int^{\infty}_{r}\overrightarrow{F}(r^{\prime})\cdot d\overrightarrow{r}^{\prime}.$$
You may assume that the potential energy at infinity, $U(\infty)$, is zero.
Exercise 2 : Use the last exercise - or \eqref{u} - to ascertain the gravitational potential energy experienced by the electron. Use the values in the text - or look them up yourself - to determine the potential energy due to gravity at $r = 1Å$. Then use \eqref{hc1} to determine the energy scale where Quantum Mechanics becomes important.
Exercise 3 : Compute the the average gravitational potential energy of the Earth in orbit around the sun. You can use Google look up whatever parameters you need to. Multiply this by the distance between the Earth and the Sun and compare your answer to hc.
Exercise 4 : Atoms often bind together to form molecules. The binding energy is similar in spirit to that of the electron. These energies are typically on the order of 1-10 eV. As we’ll see in a future episode, photons - particles of light - can be used to break molecules apart into atoms. The energy of a single photon is given in terms of its wavelength $\lambda$:
$$E = \frac{hc}{\lambda},$$
Determine the wavelength of photon required to break up molecules into atoms. Check where that wavelength is on the spectrum of light and comment on which range it can be found in.
$^{1}$ : Recall that a Newton [N] is a unit for force, and a Joule [J] is a Newton-meter [N$\cdot$m]. So the assumption here is that we will report $r$, the distance between the particles, in meters.
$^{2}$ : An electronvolt or eV is a measure of energy. It’s the electron charge e times 1 Volt. Heuristically, you can think of electrons coming out of a 9V battery has having 9 eV of kinetic energy, each. More precisely, 1 eV equals $1.6\times10^{-19}$ J of energy. 1 Joule of energy is about how much it takes to pick up a deck of playing cards from the table.
$^{3}$ : Don’t let that minus sign bother you, it’s just a convention meant to go with the whole “well” analogy. You can alternatively think of it like this: it takes a positive amount of energy - perhaps in the form of a photon - to knock the electron out of that potential energy well.
$^{4}$ : Specifically, we take $U(\infty)$ to be zero. Because $U(r)$ is defined by an integral, it can always be adjusted by an additive constant.
$^{5}$ : You might have noticed by now that applications of \eqref{hc1} give much larger numbers than expected. All this means is that Quantum Mechanics - as it applies to electromagnetism anyway - has really important effects at the scale of atoms! If you repeated this with Gravitation, as you will in the exercises, you’ll see how unimportant quantum gravity is compared to quantum electrodynamics.