Photon Transitions in Hydrogen
We continue our study of the Hydrogen atom by looking at the specific wavelengths of light it emits. The energies of the individual particles of light - the photons - emitted are then considered in terms of the previously studied product of physical parameters $hc$.
Last time we explored the constants of nature fundamental Quantum Mechanics: $h$ and $c$. In particular, we saw that combination give us a characteristic length scale for a given energy:
$$hc = 1240 \,\mathrm{eV}\cdot \mathrm{nm}.$$
By comparing this relationship to Coulomb's law of electrostatic force, we noticed that quantum effects became important when the electron was less than $0.1$ nm from the proton. Today we'll explore the relationship between $hc$ and they Hydrogen atom from a slightly different angle.
We’ve also seen that $hc$ determines the resolution of light waves by fixing a discrete number of photons with a minimum energy per wavelength of light:
$$E = \frac{hc}{\lambda}.$$
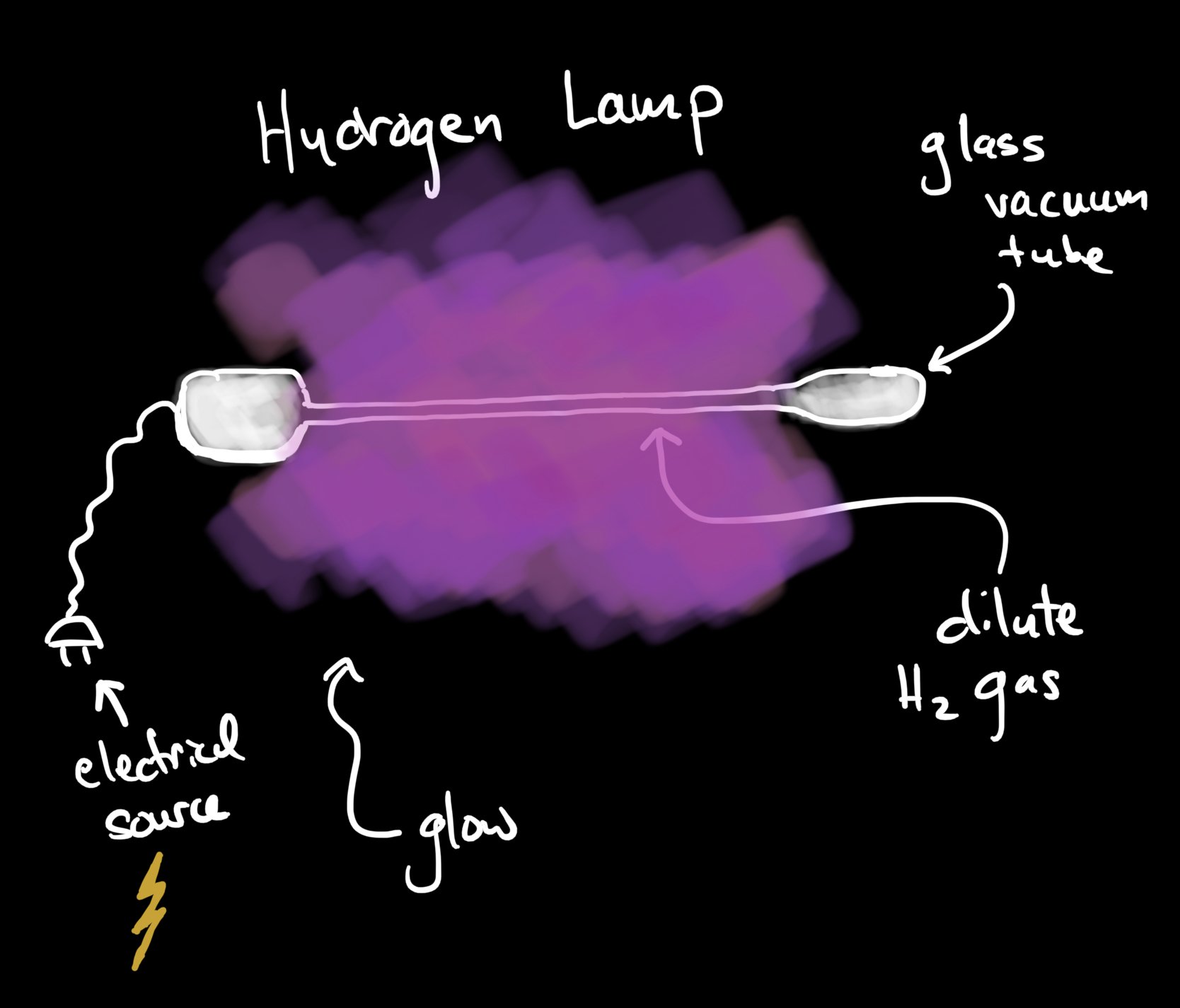
Hydrogen Lamps
Hydrogen appears in nature as a diatomic gas, meaning it comes in pairs of atoms, with two atoms joined together, H$_{2}$. Each atom contributes its own proton and electron.
In low densities, Hydrogen gas can be made into a lamp. Placed in low pressure glass tubes, exposure to electrical current causes the hydrogen gas to glow. The electric current energizes the electrons. They gain enough energy to break the diatomic bond, but not enough energy to escape the Coulomb force that binds them into atoms. They do, however, gain enough energy to reach a higher orbit. The current gives them more potential energy.
As the electrons fall back down to a lower energy, they emit particles of light or photons. In aggregate, those photons constitute the light of the lamp.
We’ve already seen that the energy of an emitted photon of wavelength $\lambda$ is given by the relationship
\begin{equation}\label{form}E = \frac{hc}{\lambda} \approx \frac{1240 \,\mathrm{eV}\cdot\mathrm{nm}}{\lambda}.\end{equation}
The Balmer Series
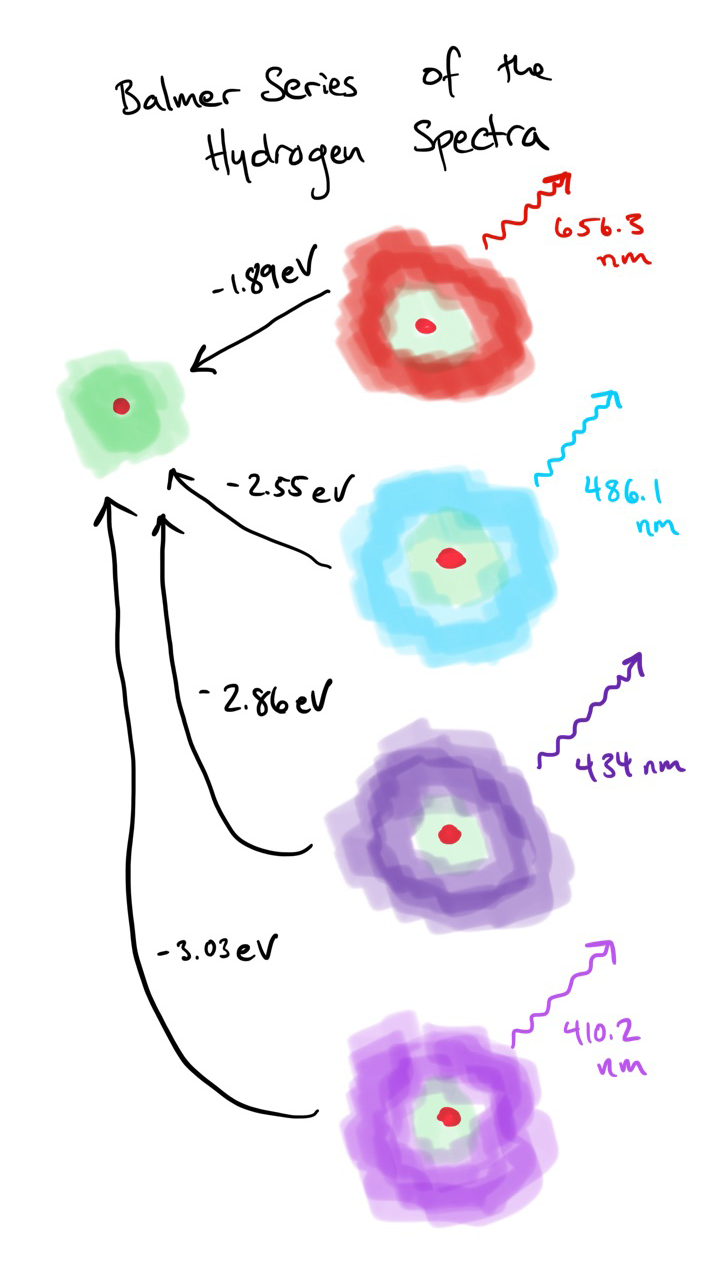
What perplexed physicists near the turn of the 20th century was the properties of the light emitted by Hydrogen. When passed through a prism, the light split not into a rainbow, but into four, distinct colors at four, very specific wavelengths. This series of four colors has come to be known as the Balmer Series:
RED : 656.3 nm : energy emitted 1.89 eV
CYAN : 486.1 nm : energy emitted 2.55 eV
BLUE-VIOLET : 434.0 nm : energy emitted 2.86 eV
VIOLET : 410.2 nm : energy emitted 3.03 eV
We now understand this discrete series of colors emitted by Hydrogen lamplight to be related to discrete or quantized energy levels of the electron in a Hydrogen atom. That is, the electrons in the atom can only exist in specific “orbits” or “energy levels”. When they drop between levels, they lose a specific amount of energy. That energy is carried away by the emission of a photon of corresponding energy.
An Empirical Formula
The discrete lines of the hydrogen spectrum corresponds to distinct energy levels of the electron inside the atom. We know this because photon energies are quantized.
Johann Balmer came up with a simple formula to describe these four hydrogen lines:
\begin{equation}\label{balmer}E(n) = (13.6 \,\mathrm{eV})\left(\frac{1}{4} - \frac{1}{n^{2}}\right).\end{equation}
You can and should readily check that the energy spectra of hydrogen correspond to the values of $n$, where
$$n = 3,4,5,\; \mathrm{and}\; 6.$$
The fact that \eqref{balmer} describes these lines in terms of whole numbers should give us pause. It strongly suggests some deeper mathematical structure built into the full description of they hydrogen atom.
We’ll get to that eventually, but for now, let’s generalize Balmer’s result a bit. The formula starts with 3, which is a peculiar number start with, especially since the first term looks like $\frac{1}{2^{2}}$.
It’s not hard to guess that this formula readily generalizes to
\begin{equation}\label{rydberg}E(n\rightarrow m) = E_{0}\left(\frac{1}{m^{3}} - \frac{1}{n^{2}}\right),\end{equation}
where $m$ and $n$ are integers.
For the hydrogen atom,
$$E_{0} = 13.6\; \mathrm{eV}.$$
In there exercises you can and should plug in different values for $m$ to understand why Balmer’s series starts with $n=3$.
Exercises
Exercise One
Work out a table for all values of \eqref{rydberg}, having $m$ and $n$ range from 1 to 10. You’ll probably want to use a spreadsheet for this. Note that this matrix is antisymmetric. These are the transitions between electron energy states for the Hydrogen atom.
Exercise Two
We’ve been talking about energy differences so far. What does this mean for the actual energies of the physical states of the hydrogen atom? You should be able to write them down for $n=1$ to $10$ now, using your results from exercise one.
Exercise Three
Balmer’s formula is an empirical one. That means it was merely invented to match the data. There are lots of ways to attempt to recreate Balmer’s work. A reasonable place to start is to fit a graph to the data points.
Assume there’s a function $f(x)$ that has four data points given by $1.89,2.55,2.86$ and $3.03$. Make a scatter plot from these points and try to fit a trend line.
Clearly a linear fit isn’t the right solution.
Next try plot a bunch of different monomial functions for $f(x)$:
$$f(x) = x^{m},$$
you might use that spreadsheet program to evaluate $f(x)$ for various values of $x$, from say $1$ to $10$.
Which value of $m$ fits data best? What is it about the shape of the curve that led you to this decision? If it helps you can pull out a ruler and a pen and do this with a sheet of paper.
Further Reading
For details on the inner workings of atoms, check out our discussions of the atomic nucleus and the electron cloud, from the Field Guide to Particle Physics.
Two great - if uncommon - textbooks on Quantum Mechanics include J.J. Sakurai’s Modern Quantum Mechanics and Gordon Baym’s Lectures on Quantum Mechanics.
Check out NASA’s tour of the electromagnetic spectrum, starting with visible light.